The pendulum used in my wooden clocks is in technical terms
a simple pendulum, in essence, a mass suspended from a fixed point so that it
can swing back and forth under the influence of gravity.
Real pendulums have
the mass fixed to the end of the pendulum rod so the actual centre of the mass
of the whole pendulum is, in reality, a little closer to the pivot as shown in
the diagrams below because the rod itself has some mass.
The formulae for calculating the period of swing is -
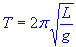
What is probably more useful is to work out the Length to
the centre of mass so that we can set the initial length of the pendulum, to do that use-
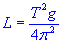
where T = Period of oscillation, i.e. time to swing back and
forth.
L = Length from Pivot to the centre of mass.
g = Acceleration due to Gravity meters/second^2 (9.81m/s^2)
Pi (π) = 3.142
L = Length from Pivot to the centre of mass.
g = Acceleration due to Gravity meters/second^2 (9.81m/s^2)
Pi (π) = 3.142
For most of my clocks I use a 2 seconds pendulum i.e. the
time to swing back and forth is 2 seconds.
To work out the length of the pendulum from the pivot point to
its centre of mass use above formula.
L=2²*9.81÷(4*3.142²) =
0.993 meters
For a 1 second pendulum used on Clocks 9,12 and 14
L=1²*9.81÷(4*3.142²) = 0.248meters
For a 1 second pendulum used on Clocks 9,12 and 14
L=1²*9.81÷(4*3.142²) = 0.248meters
Links to Useful Websites:
Useful Formulae for Simple Pendulum calcs
History of the Pendulum use in clockshttp://en.wikipedia.org/wiki/Pendulum_clock#Atmospheric_drag
